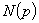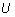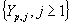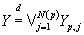| Article ID: | iaor1992386 |
| Country: | United States |
| Volume: | 7 |
| Start Page Number: | 191 |
| End Page Number: | 218 |
| Publication Date: | Aug 1991 |
| Journal: | Stochastic Models |
| Authors: | Rachev S.T., Resnick S. |
| Keywords: | stochastic processes |
The authors consider a stability property for