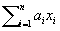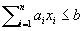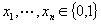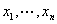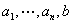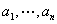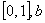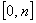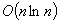| Article ID: | iaor19911682 |
| Country: | Germany |
| Volume: | 35 |
| Start Page Number: | 113 |
| End Page Number: | 149 |
| Publication Date: | Feb 1991 |
| Journal: | Mathematical Methods of Operations Research (Heidelberg) |
| Authors: | Borgwardt K.H., Tremel B. |
This paper deals with the quality of approximative solutions for the Subset-Sum-Maximization-Problem. Maximize