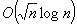| Article ID: | iaor19941942 |
| Country: | United States |
| Volume: | 19 |
| Issue: | 1 |
| Start Page Number: | 38 |
| End Page Number: | 52 |
| Publication Date: | Feb 1994 |
| Journal: | Mathematics of Operations Research |
| Authors: | Ye Yinyu |
| Keywords: | interior point methods |
The paper proposes an approach based on interior-point algorithms for linear programming (LP). It shows that the algorithm solves a class of LP problems in strongly polynomial time,