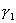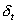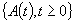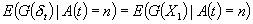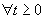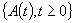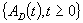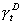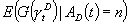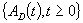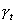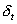Let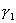 
and 
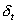denote the residual life at t and current life at t, respectively, of a renewal process 
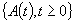, with 
and sequence of interarrival times. The authors prove that, given a function G, under mild conditions, as long as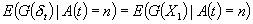 
, 
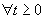holds for a single positive integer n, then 
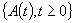is a Poisson process. On the other hand, for a delayed renewal process 
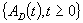with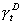 
the residual life at t, they find that for some fixed positive integer n, if 
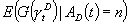is independent of t, then 
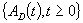is an arbitrarily delayed Poisson process. The authors also give some corresponding results about characterizing the common distribution function F of the interarrival times to be geometric when F is discrete. Finally, they obtain some characterization results based on the total life or independence of 
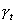and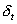 
.
