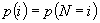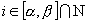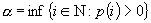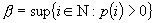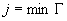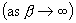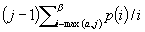| Article ID: | iaor19932463 |
| Country: | Germany |
| Volume: | 37 |
| Issue: | 1 |
| Start Page Number: | 97 |
| End Page Number: | 106 |
| Publication Date: | Jan 1993 |
| Journal: | Mathematical Methods of Operations Research (Heidelberg) |
| Authors: | Lethinen A. |
| Keywords: | programming: dynamic |
The secretary problem with a known prior distribution of the number of candidates is considered. If