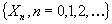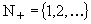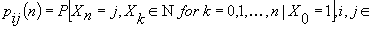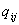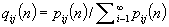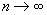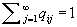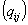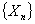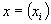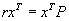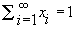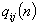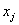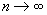Let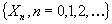 
be a transient Markov chain which, when restricted to the state space 
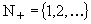, is governed by an irreducible, aperiodic and strictly substochastic matrix 
, and let 
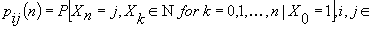. The prime concern of this paper is conditions for the existence of the limits, 
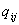say, of 
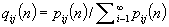as 
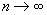. If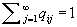 
, the distribution 
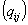is called the quasi-stationary distribution of 
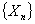and has considerable practical importance. It will be shown that, under some conditions, if a non-negative non-trivial vector 
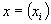satisfying 
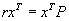and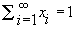 
exists, where r is the convergence norm of P, i.e. r=R-1 and 
, and T denotes transpose, then it is unique, positive elementwise, and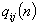 
necessarily converge to 
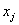as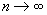 
. Unlike existing results in the literature, the present results can be applied even to the R-null and R-transient cases. Finally, an application to a left-continuous random walk whose governing substochastic matrix is R-transient is discussed to demonstrate the usefulness of the results.
